Harmonic Analysis

This example demonstrates how to perform harmonic analysis on a signal using the harmonic_analysis function.

First, we import the required modules.

from __future__ import annotations

import pathlib

import matplotlib.pyplot
import netCDF4
import pyfes

The distribution contains a time series fes_tide_time_series.nc that will be used in this help.

SIGNAL = (pathlib.Path().absolute().parent / 'tests' / 'python' / 'dataset' /
          'fes_tide_time_series.nc')

Load the signal.

with netCDF4.Dataset(SIGNAL, 'r') as ds:  # type: ignore
    time = (ds.variables['time'][:]).astype('datetime64[us]')
    h = ds['ocean'][:] * 1e-2  # cm to m

Then, we will create an instance of a pyfes.WaveTable object:

wt = pyfes.WaveTable()

By default, all components known by this object are loaded into memory. The list of components known by this object can be retrieved using the pyfes.constituents.known() method.

print(pyfes.constituents.known())

['Mm', 'Mf', 'Mtm', 'Msqm', '2Q1', 'Sigma1', 'Q1', 'Rho1', 'O1', 'MP1', 'M11', 'M12', 'M13', 'Chi1', 'Pi1', 'P1', 'S1', 'K1', 'Psi1', 'Phi1', 'Theta1', 'J1', 'OO1', 'MNS2', 'Eps2', '2N2', 'Mu2', '2MS2', 'N2', 'Nu2', 'M2', 'MKS2', 'Lambda2', 'L2', '2MN2', 'T2', 'S2', 'R2', 'K2', 'MSN2', 'Eta2', '2SM2', 'MO3', '2MK3', 'M3', 'MK3', 'N4', 'MN4', 'M4', 'SN4', 'MS4', 'MK4', 'S4', 'SK4', 'R4', '2MN6', 'M6', 'MSN6', '2MS6', '2MK6', '2SM6', 'MSK6', 'S6', 'M8', 'MSf', 'Ssa', 'Sa', 'A5', 'Sa1', 'Sta', 'Mm2', 'Mm1', 'Mf1', 'Mf2', 'M0']

If you want to restrict the analysis to only a few components, you must provide a list to the constructor in order to specify the waves to be analyzed.

wt = pyfes.WaveTable([
    'Mm', 'Mf', 'Mtm', 'Msqm', '2Q1', 'Sigma1', 'Q1', 'Rho1', 'O1', 'MP1',
    'M11', 'M12', 'M13', 'Chi1', 'Pi1', 'P1', 'S1', 'K1', 'Psi1', 'Phi1',
    'Theta1', 'J1', 'OO1', 'MNS2', 'Eps2', '2N2', 'Mu2', '2MS2', 'N2', 'Nu2',
    'M2', 'MKS2', 'Lambda2', 'L2', '2MN2', 'T2', 'S2', 'R2', 'K2', 'MSN2',
    'Eta2', '2SM2', 'MO3', '2MK3', 'M3', 'MK3', 'N4', 'MN4', 'M4', 'SN4',
    'MS4', 'MK4', 'S4', 'SK4', 'R4', '2MN6', 'M6', 'MSN6', '2MS6', '2MK6',
    '2SM6', 'MSK6', 'S6', 'M8', 'MSf', 'Ssa', 'Sa'
])

The pyfes.WaveTable.keys method allows to retrieve the list of waves defined during the construction of the object.

print(wt.keys())

['Mm', 'Mf', 'Mtm', 'Msqm', '2Q1', 'Sigma1', 'Q1', 'Rho1', 'O1', 'MP1', 'M11', 'M12', 'M13', 'Chi1', 'Pi1', 'P1', 'S1', 'K1', 'Psi1', 'Phi1', 'Theta1', 'J1', 'OO1', 'MNS2', 'Eps2', '2N2', 'Mu2', '2MS2', 'N2', 'Nu2', 'M2', 'MKS2', 'Lambda2', 'L2', '2MN2', 'T2', 'S2', 'R2', 'K2', 'MSN2', 'Eta2', '2SM2', 'MO3', '2MK3', 'M3', 'MK3', 'N4', 'MN4', 'M4', 'SN4', 'MS4', 'MK4', 'S4', 'SK4', 'R4', '2MN6', 'M6', 'MSN6', '2MS6', '2MK6', '2SM6', 'MSK6', 'S6', 'M8', 'MSf', 'Ssa', 'Sa']

In order to compute the astronomical angular argument, the leap seconds must be known. The leap seconds can be obtained using the pyfes.get_leap_seconds() function.

leap_seconds = pyfes.get_leap_seconds(time)

The different nodal corrections are then calculated from the time series to be analyzed:

f, vu = wt.compute_nodal_modulations(time, leap_seconds)

These coefficients are used by harmonic analysis to determine the properties of the different tidal waves defined during the construction of the instance.

w = wt.harmonic_analysis(h, f, vu)

This result can then be used to determine a tidal height for the analyzed time series:

h_tide = wt.tide_from_tide_series(time, leap_seconds, w)

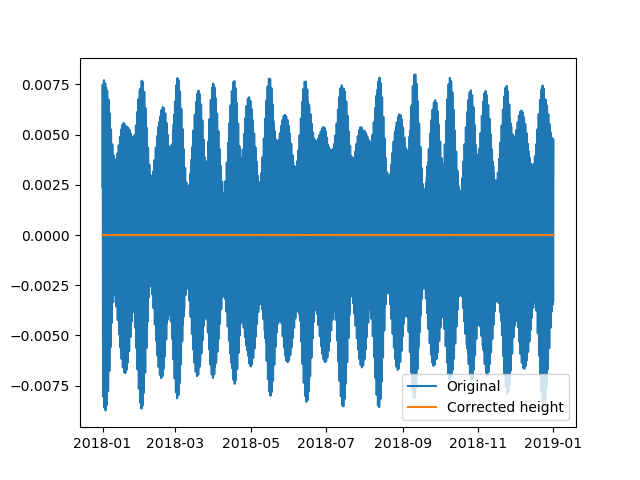
Finally, we can visualize the original signal’s height adjusted by the tidal height derived from harmonic analysis. The outcome is zero because the analyzed signal originates from the tidal model using identical waves as those in the harmonic analysis.

matplotlib.pyplot.plot(time, h, label='Original')
matplotlib.pyplot.plot(time, h - h_tide, label='Corrected height')
matplotlib.pyplot.legend()

<matplotlib.legend.Legend object at 0x7f523075d210>